|
Author
|
Topic: Lunar Altitudes for Longitude
|
bterhart
|
 posted April 05, 2018 06:09 PM
posted April 05, 2018 06:09 PM


Hi,
I'm trying to determine longitudes using Bennett's revised lunar altitude methodology. It's referenced in the Cel Nav textbook and involves an arithmetical approximation of the iterative method mentioned in the Cel Nav and Emergency Nav books.
The method is attractive but I cannot get anything close to reasonable. Using the example data in the Emergency Nav book for a two-star fix and moon-sight, I get wildly different results. I can, however, easily reproduce Bennett's example in his 2006 paper.
Bennett's revised method and the iterative method referred to above both rely on the same principle (at least as I understand it!). That is, there is a pair of values for watch error and longitude that correspond to a lunar intercept of 0.
Does anyone have a worked example of Bennett's method and a matching interative solution that I could go over?
TIA,
Bert
From: Nanaimo
|
|
David Burch
|
 posted April 05, 2018 06:22 PM
posted April 05, 2018 06:22 PM


I do not know of that specific example, maybe someone else does.
But if you care to post the data we can try to work it to see what we get. we need Ho and WT for a series of moon sights to the east or west, plus either two stars sights or a known Lat. The WT of all the sights have to be on the same watch, but we do not need error or ZD. we also need a DR position, can be off by some reasonable amount, ie couple hundred miles.
From: Starpath, Seattle, WA
|
|
bterhart
|
 posted April 06, 2018 06:59 PM
posted April 06, 2018 06:59 PM


From Bennett's paper:


This method appears to be an arithmetical shortcut to a number of plotting iterations. Seems reasonable and attractive : )
I thought to test this method with some known data. Since an unknown watch error introduces an error in longitude, introducing a known longitude error should allow us to determine the correlated watch error. Let's assume that:
Our real location at 13 00 00 UTC 1 Jan 2018 is:
Lat 49 12.0N
Long 123 35.0W
Our assumed (DR) location has us slightly to the West at:
Lat 49 12.0N
Long 123 45.0W
In other words, we have a watch error of some magnitude that has us to the West.
From http://aa.usno.navy.mil/data/docs/celnavtable.php
Hc and Zn for the Moon at 13 00 00 UTC at Lat 49 12.0N Long 123 35.0W is:
Hc = 21 50.3
ZN = 275.6
* Note that as required, the Moon's azimuth is almost exactly due West.
Hc in this case is our Ho. We're at this location exactly so this is what we would observe. We think however, that we're at 123 45.0W due to a watch error. A sight reduction for this Longitude (same Lat, same time) yields:
Hc = 21 56.8
Zn = 275.4
So far so good. Our intercept, a is:
a= Ho - Hc = 21 50.3 - 21 56.8
a= 6.5 A
We can check this quickly as our intercept should be equal to DCosLAT = 10Cos49.2=6.5
Using Bennet's methodology, let Ip=0, If=DR intercept, Lf=DR Longitude, Is=intercept at time+20min, Ls=Longitude+5deg and solve for Wp and Lp where Wp and Lp are the correlated watch error and Longitude respectively.
At 13 20 00 UTC with
LAT 49 12.0
LONG 128 45.0
Moon Hc and Zn is:
Hc = 22 05.5
Zn = 275.3
Therefore Is = Ho - Hc = 21 50.3 - 22 05.5
Is = 15.2 A
Solving for F:
F = Is/(Is - If)
F = -15.2/[-15.2-(-6.5)]
F = 15.2/8.7
Solving for Wp:
Wp = 20 - F20= -14.9425
Our watch error is therefore 14min 56sec fast.
Solving for Lp:
Lp = Ls-F5=128 45.0 - (15.2/8.7)5 = 120 00.9W
And therein lies the rub: We haven't come close to our correct Longitude of 123 35.0W
We have a known position, a known longitude error, a known Ho and correct values for Hc. Why the huge error in Longitude?
According to Bennett, one can check the Wp and Lp values by computing a Moon intercept at that time and longitude. That intercept should be zero. Thus, for Wp and Lp, Hc is calculated at:
UTC = 12 45 04
Lat = 49 12.0N
Long = 120 00.9W
Hc = 21 50.4
a = Ho - Hc = 21 50.3 - 21 50.3 = 0.0 A
a = 0 is equivalent to iterating a number of increasingly accurate plots and guesses to the correct watch error.
Apparently, we've got the methodology correct. Either I don't understand it very well at all (very possible!) , I've made mistake along the way (wouldn't be the first time), or the method leaves something to be desired...
Any help clearing this up would be appreciated!
Thanks,
Bert
From: Nanaimo
|
|
bterhart
|
 posted April 06, 2018 07:05 PM
posted April 06, 2018 07:05 PM


To view the images in the above post, right click and open in a new tab.
From: Nanaimo
|
|
David Burch
|
 posted April 06, 2018 10:07 PM
posted April 06, 2018 10:07 PM


Hi Bert, I am sorry i was not clear. All we need is an example that he works and gets and answer to, but you cannot reproduce it. ( ie we need his example that you cannot confirm, not a new one.) We cannot get into his method here, but we can check one that he presents to see if we get the same answer with our own methods,
It is not our policy to study procedures from other books or papers, but we can solve specific problems to see if the answer they give is correct.
-----
On another topic, we do have an N(x) digital solution for you. We will package this up shortly and post a link here.
From: Starpath, Seattle, WA
|
|
bterhart
|
 posted April 07, 2018 08:29 AM
posted April 07, 2018 08:29 AM


Hi David,
Nothing to apologize for David. I'll rework his example and the example I presented using your methods and post the results here. That should be enough for anyone coming across the thread to get an understanding of the issues.
Thanks again,
Bert
From: Nanaimo
|
|
bterhart
|
 posted April 09, 2018 11:19 AM
posted April 09, 2018 11:19 AM


Consider the following work as a comparison between the iterative lunar altitudes method of regaining UTC vs the interpolation method of Bennett. The iterative method is discussed or referenced in both the Cel Nav text and the Emergency Nav text. Both the iterative and interpolation methods are based on the same principle: There exists a combination of watch error and longitude that gives an intercept of zero for a Moon sight taken some time near a reasonable fix (although uncertain in longitude) has been obtained. If the sight of the Moon was taken with it bears nearly due East or West, the resulting LOP will be nearly vertical and therefore an accurate representation (within observational limits) of longitude albeit in error directly proportional to watch error. The calculated watch error and/or correlated longitude enable one to regain UTC within the limits of the observations and the method itself.
That said, I took the example given in Bennett's paper and re-worked the example using the interpolation method. The results are as follows:
1. A round of star-sights were taken as follows (height of eye 3.0m, IC =2.0', Course 050T, Speed 6.0kn, assumed position 41N 15W):
22 October 2005
Hamal 06 54 25.3 Hs 17 30.6
Procyon 06 55 07.0 Hs 53 48.2
Alioth 06 55 33.7 Hs 41 15.8
Fix at 06 55 00 41 15.5'N; 15 36.0'W
2. At 09 07 55.0, an upper limb of the moon was observed at Hs 37 09.7'
A running fix (RFIX) brought up from the 06 55 fix gives a position at 09 08 of:
41 24.1'N ; 15 22.5'W
Careful plotting of the Moon's LOP from the 09 08 RFIX shows us 3.2 min of longitude west. Given that when the Moon is East of our position, watch error is fast and that 1min of longitude is 2minutes of time, our clock is 6min 24sec FAST.
3 iterations of star fixes at corrected times and careful plotting of the RFIXs and Moon's LOP eventually yields an intercept for the Moon of zero at a watch error of 4min 55sec fast and a position of 41 23.7'N 14 08.2'W. This is very much different than Bennett's solution to the same problem (5min 47sec fast, 41 24.1'N ; 13 56.0'W). The problem is that both solutions produce an intercept of zero and, theoretically, both are correct.
I tried using Bennett's method on test data and in no instance could I produce reasonable results. On the same test data, the iterative approach produced accurate results.
I would conclude, then, that the interative method is a much superior method of regaining some semblance of UTC when using lunar altitudes in this manner.
For the sake of brevity and (I hope!) clarity, my plotted and computed solutions are available for the asking ([email protected]).
Bert
From: Nanaimo
|
|
David Burch
|
 posted April 09, 2018 01:29 PM
posted April 09, 2018 01:29 PM


Bert,
We can work this example out by standard procedures, but this is not what we recommend for WE by lunar altitude method. I would doubt, without even working on it more, that this would not yield good results.
You have a star fix (that should give correct Lat, regardless of WE) and then over 2 hours later you do a moon sight. The uncertainty in the DR over this run would likely wipe out any time accuracy completely. We need moon and star sights to essentially simultaneous. that is, you rotate sights, star star moon star star moon star star moon then you plot all vs time and find equivalent simultaneous values.
As i understand it, you analyze this data and conclude the WE is 4min 55sec fast, but when Bennett analyzes the same data he concludes the WE is 5min 47sec fast.
Is that correct?
With that said, if still interested, we can work this example. Accurate or not, there should be a right answer.
==========================================
----- note the moon is west enough at moon sight time, ie just 7º off of west, but the sun was then up and no stars to do. so this must be why the times are offset so much.
code:
Celestial Navigation Data for 2005 Oct 22 at 9:07:55 UT
For Assumed Position: Latitude N 41 24.1
Longitude W 15 22.5
Almanac Data | Altitude Corrections
Object GHA Dec Hc Zn | Refr SD PA Sum
o ' o ' o ' o | ' ' ' '
SUN 320 51.7 S11 08.5 +17 25.4 123.1 | -3.2 16.1 0.1 13.1
MOON 78 47.6 N28 32.3 +37 38.9 277.1 | -1.3 15.3 44.4 58.4
At his star sight time, the moon was 17º off of west
code:
Celestial Navigation Data for 2005 Oct 22 at 6:55:00 UT
For Assumed Position: Latitude N 41 15.5
Longitude W 15 36.0
Almanac Data | Altitude Corrections
Object GHA Dec Hc Zn | Refr SD PA Sum
o ' o ' o ' o | ' ' ' '
SUN 287 37.8 S11 06.6 - 5 47.6 99.7 | --- --- --- ---
MOON 46 46.9 N28 29.9 +61 37.5 253.2 | -0.6 15.4 26.8 41.6
=====
so this could be a realist challenge, namely you worry about your time but do not have the ideal conditions for lunar altitude... and for some reason do not want to do a full lunar distance measurement. (sun moon is too bar apart for this, but stars would work.)
From: Starpath, Seattle, WA
|
|
bterhart
|
 posted April 09, 2018 04:35 PM
posted April 09, 2018 04:35 PM


Hi Dave,
Yes, that's right: The two different analysis yield two different correct results. When I say correct, I mean that the moon intercept at the correct time = 0. That's the problem with the method: There is no unique answer. There are other combinations of watch error and longitude that give a Moon intercept of zero.
The iterative method outlined in your texts zeroes in on the watch error and longitude that most closely recaptures UTC. At least that's my inference from comparing the two. Your other observations (DR errors, etc) I would most heartily agree with ![[Smile]](smile.gif) . .
I computed star sight fixes using StarPath and corrected times, then plotted the RFIXs. I would be interested in a worked example for sure. At the very least it would be a check of what I've done and my understanding. I'll check my own work again to make sure I've not made an error somewhere along the way.
Thanks and much appreciated!
Bert
From: Nanaimo
|
|
bterhart
|
 posted April 09, 2018 06:50 PM
posted April 09, 2018 06:50 PM


Rechecked my work and found an arithmetical error when plotting in my first set of iterations (RFIX Long of 13 46.1W as opposed to 13 45.3'W). Final WE and Longitude recalculated/replotted is 5min 04sec Fast and Long 14 06.0'W. This is a difference of only 9sec which would seem reasonable given the small difference in RFIX Long partway through the interations (0.8').
The overall conclusion is the same: There is not a unique set of WE and corresponding Long as this WE and Long still give a Moon intercept of zero. The two different methods each converge on a solution. The solutions, however, are different.
IE:For an upper limb shot of the Moon with Hs 37 09.7, height of eye 3.0m, and IC +2.0 at:
09 02 51 ; 41 23.9N ; 14 06.0W : a = 0 Zn=277.2 WE 5min 04sec FAST (Corrected as above)
09 03 00 ; 41 23.9N ; 14 08.2W : a = 0 Zn=277.2 WE 4min 55sec FAST (With RFIX error as per my other post)
09 02 08 ; 41 24.1N ; 13 55.7W : a = 0 Zn=277.2 WE 5min 47sec FAST (Bennett)
I still think the iterative approach is better. Perhaps we're just looking at the limits of the methodology...
Thanks again Dave!
Bert
From: Nanaimo
|
|
David Burch
|
 posted April 09, 2018 10:36 PM
posted April 09, 2018 10:36 PM


OK. Excellent.
Congratulations on tracking this down.
I must note that all these solutions average 5 m 15s ±30s... which is the best you can hope for. again, in a real case, you could not likely get ± 30s with such a running fix, as it would wipe out the accuracy needed.
A good way to test this is have a friend off set your watch by some few minutes and then find a moon due east or west in the back yard and with a leveled mirror artificial horizon just test it.
the lat of the star fix is will tell if the mirror is level, then see if you can find the WE.
or even more easily but not quite as good a practical test, go to starpath.com/usno and find a moon to the E or W and then take some stars from that list at that time, use Hc for Ho in all cases and sight reduce with a known 3 or 4 minute off set and see if you can pull it back out. I think that should work.
all these Lon from the moon sights need to be very accurate.
From: Starpath, Seattle, WA
|
|
bterhart
|
 posted April 10, 2018 07:53 AM
posted April 10, 2018 07:53 AM


Hi Dave,
Trying Bennett's method with starpath.com/usno test data led to all this. I'll repeat the same test using the iterative method used here and post here. I'll bet I can pull out the induced error within reasonable limits : )
From: Nanaimo
|
|
bterhart
|
 posted April 10, 2018 11:36 AM
posted April 10, 2018 11:36 AM


Hi Dave,
To show how well the interative method can work, I choose the example I started this thread with. Specically:
UTC Jan 1, 2018 13:00:00
DR 46 12.0N 123 35.0W
WE 4min FAST
Selected stars Procyon, Vega, Spica, and the Moon were 'observed' at 13:04:00 WT and Ho calculated from http://starpath.com/usno data using the real UTC (13:00:00).
A single round of careful plotting produced a Moon LOP 2' East of our DR and therefore our first crack at an estimate of WE gave us 4min FAST (recall that 1' of long = 2min of watch time). I didn't bother with plotting at a larger scale ![[Smile]](smile.gif) . .
The following image shows the plotting work:
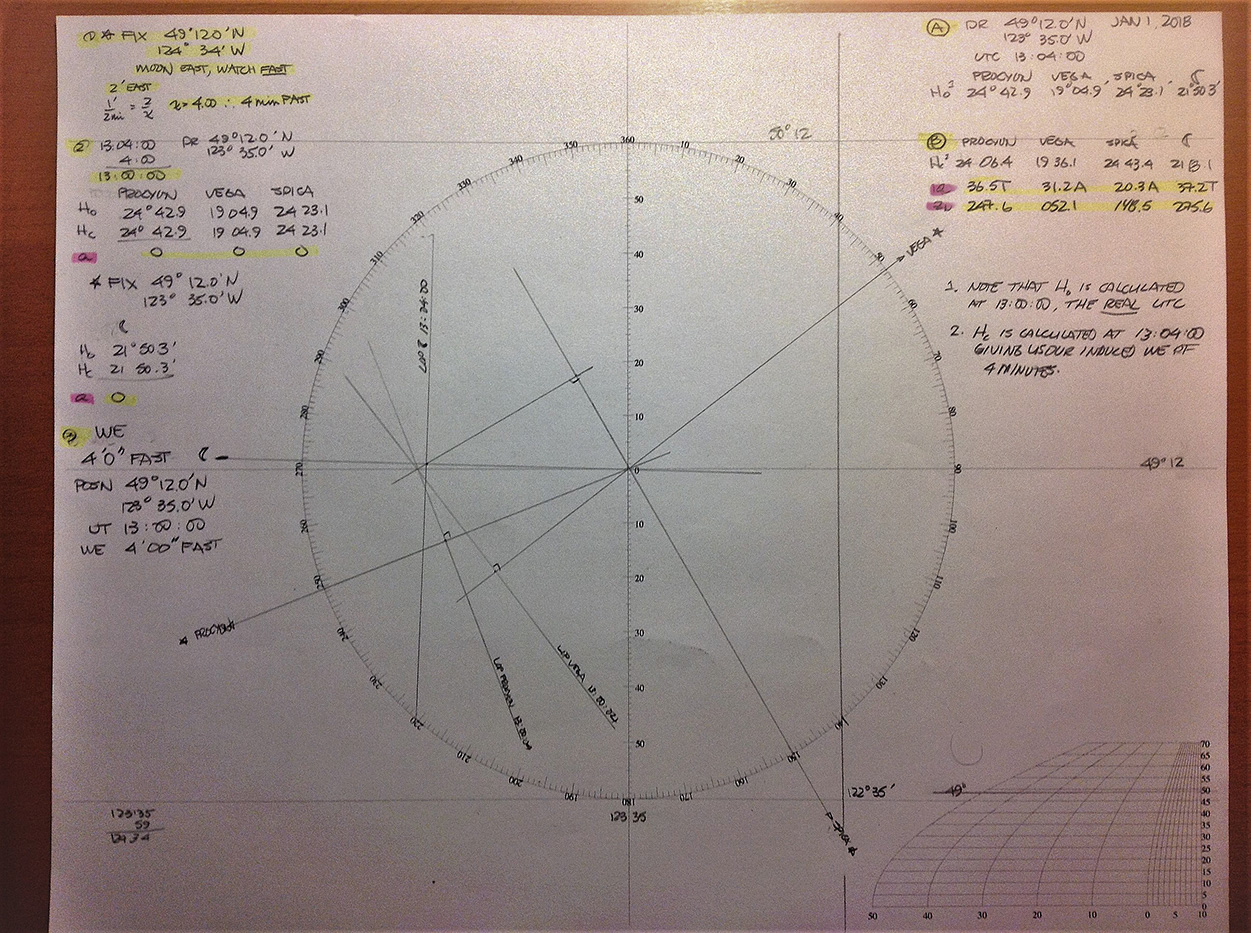
AS you predicted, using exact data one can pull out the introduced WE within limits.
Thanks again for your time Dave. Much appreciated!
Bert
From: Nanaimo
|
|
|









