|
Author
|
Topic: Question 5-5
|
Michael Mitgang
|
 posted January 25, 2005 09:41 PM
posted January 25, 2005 09:41 PM


I get 48 degrees, 19.7 N rather than 19.9 N (which according to my calculations is approximately 400 yards off. I did it both on the computer & using paper charts. I got the longitude correct. Is this within a reasonable degree of accuracy?
From: Menlo Park, CA
|
|
David Burch
|
 posted January 27, 2005 01:53 PM
posted January 27, 2005 01:53 PM


I will have to work this one again to see if I agree with the given answer. Someone else solved these originally.
However, in the meantime, the general way one would evaluate your question would be in terms of percentages... and of course the chart scale you are working on.
Without even looking at the problem, i would propose that one should be able to do at least 5% accuracy in the overall process assuming all of your inputs are correct.
So is 400 yards big or small compared to the total distance traveled in this problem? ie, what percent is 400 yard of the total distance traveled?
The answer to Part B says we travled 2.8 nmi, so we have 400 yd/(2.8 x 2000 yd) = 0.071, which is 7.1%. So, again, without doing this problem, i would have to conclude that 400 yd in this case is too large an error. Either the printed answer is wrong or you have a mistake some where.
On the other hand, if you are sailing in an area with unknown current, or you have not calibrated your compass or your log, or you have not been careful about the heading while dirving, etc... then we have got to bump up the expected uncertainly level. And with any of these present, 7% may not be so bad.
In this particular problem, i would assume that one of us is wrong.... real navigation in this part of the world however, is much trickier as there are indeed large and hard to predict tidal currents present. They are not mentioned, though, in this exericse.
From: Starpath, Seattle, WA
|
|
Michael Mitgang
|
 posted January 28, 2005 05:01 PM
posted January 28, 2005 05:01 PM


Thanks, I look foward to seeing your solution.
From: Menlo Park, CA
|
|
David Burch
|
 posted February 04, 2005 05:01 PM
posted February 04, 2005 05:01 PM


Well I must first apologize for the delay in getting back. I thought this was one we could work out numerically and therefore easily resolved. But that is not the case... and it turns out to be an excellent question raising some good points.
First, this is a running fix using *different* targets. To analyze this numerically as we have done for one target rfix is not possible. This type is much more involved and not really worth it without a programmed calculator.
So we are left with plotting solution and an e-chart solution. I re-plotted and got 48° 19.73'N, 123° 49.18'W, which in fact agrees with your answer, implying the printed answer is off some.
Next, as shown below, i worked this out on the e-chart and got 19.77' N and 49.11' W, which is 0.04' of lat or 240 feet off, which is OK, meaning my plot agrees with my e-chart solution, both of which show the printed answer is wrong, and your answer is right.
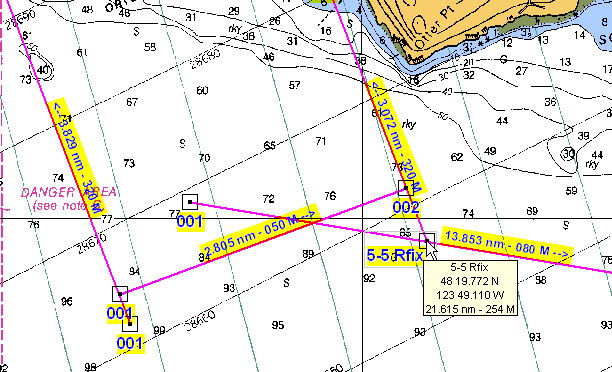
So we will fix the answer in the notes for the next printing.
More important, however, in redoing this problem, it became clear that my quick analysis of what might be expected as an error (as outlined above as roughly 5% of distance run, etc) is not necessarily the best analysis for this particular fix.
We have a potentially overriding issue in this one, in that our data itself may have uncertainties that might dominate the conclusions.
In particular, the Race Rocks light at the time of the second sight was about 2.2 nmi off. Now the question arises as to how much would the running fix be off if our measured bearing was off 1° — and that is a optimistic assumption of our precision.
Remember the very useful rule used throughout the course, that a 6° right triangle has ratio of sides equal to 1:10, or 0.10, and that this can scale down forever and up to about 18°, in which case the sides scale as 3:10 or 0.3. So 3° = 0.05 and 1° = 0.017.
In other words, if we move the Race Rocks bearing line from 080M to 081M, at 2.2 miles off we would shift the fix intersection about 0.017 x 2.2 nm = 0.017 x 2.2 x 2000 yards = 75 yards.
For every degree of bearing error we have, we introduce an error of 75 yards when the target is 2.2 nm away. This is why we choose close targets whenever possible. The closer the target, the smaller the fix error is in response to the bearing error.
In this particular problem, the 5% seems to hold up ok. We can usually get hand held bearings to within a few degrees at worst, so even at 3° uncertainty, we have to conclude that 400 yards is too large an error on this type of fix.
We have done this numerically here, but remember you can always just plot a fix with one bearing line then tweak it a degree or two and see how the fix changes. It always pays to keep in mind these uncertainties when evaluating your fixes.
A long answer to a short, but important question! Do not hesitate to raise questions.
It is fundamentally important to know and believe that your navigation analysis is correct. Discrepancies should be resolved, not accepted. The value of this type of course is to help establish what can and should be expected from your navigation.
There was a woman mate in the pilothouse of the Exxon Valdez who told the officer in charge two different times that there was a light on the horizon that was on the wrong side of the bow. That was a piece of navigation information that was tragically not pursued. We cannot accept things that do not make sense. It is usually a sign that we are not confident with our knowledge.
From: Starpath, Seattle, WA
|
|
Michael Mitgang
|
 posted February 04, 2005 09:37 PM
posted February 04, 2005 09:37 PM


Thanks for looking at both these questions.
From: Menlo Park, CA
|
|
|









